12. The vertices of the strong interaction
When we deal with electric charge we recognise two types, positive (+) and negative (-). We learn that positives and negatives attract and like charges repel.
Quarks carry a kind of non-electric charge called colour. This charge has three types that are labelled red (r), green (g) and blue (b). The grouping of r+g+b is an attractive grouping but pairs of colour repel. For each colour there is an anti-colour i.e. antired ( ), antigreen ( ) and antiblue ( ). Colour anti-colour pairs attract in the same way that the combination red+green+blue is a neutral cohesive combination. This means that red and antired attract each other, green and antigreen attract, blue and antiblue attract; but red and antiblue repel, blue and antired repel etc.
It is these rules of colour combination that drive the quarks to combine into groupings of three and quark antiquark pairs. In the groupings of three quarks (baryons), there will be red, green and blue charges in combination. In the quark antiquark pairs (mesons), there will be colour and corresponding anticolour charges in combination.
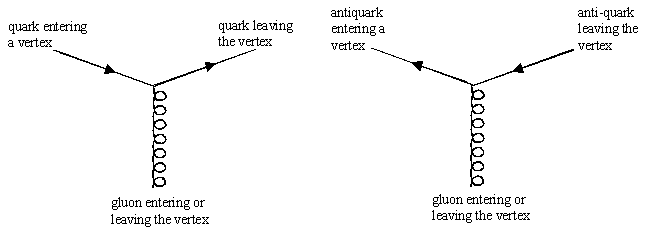
The gluon can be regarded as a pathway through which colour charge is exchanged between quarks and antiquarks. As with the electromagnetic and weak vertices there will be a fixed number of orientations of the three arms but the quark gluon vertices could also show colour flow as quarks interact.
The quark gluon vertices:
Note: each of the vertices shown below should be read from left to right. The left hand side represents BEFORE and the right hand side represents AFTER

The first vertex produces the following set of combinations:

When following a reaction it is worthwhile tracking the colour flow. Whenever a quark line meets a gluon, the colour must flow from the quark line onto the gluon. Tracking colour in this way, and recognising that quarks like to group into rgb coloured trios and colour-same anticolour pairs enables one to see why the occurrence of quark-antiquark pairs in nucleons leads to the appearance of pions.
Back to electromagnetic vertices back to the index page Next page: Examples of the use of Feynman vertices to describe processes